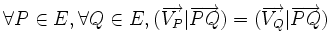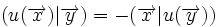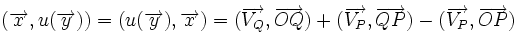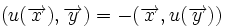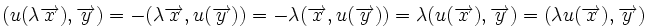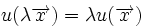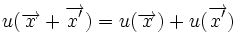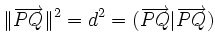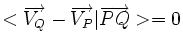- Champ equiprojectif
-
Champ équiprojectif
Dans un espace affine euclidien E, un champ de vecteurs
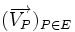 est équiprojectif si :
est équiprojectif si :où
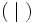 désigne le produit scalaire.
désigne le produit scalaire.Il existe alors un endomorphisme antisymétrique u tel que :
-
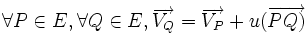 .
.
Sommaire
Démonstration
Antisymétrie
Soit O un point arbitraire de E. Pour tout vecteur
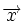 , il existe un unique point P tel que
, il existe un unique point P tel que 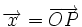 et on définit u par
et on définit u par 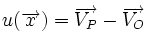 .
.Montrons que, pour tous vecteurs
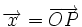 et
et 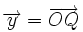 , on a :
, on a :ce qui prouve l'antisymétrie de u.
On a en effet :
-
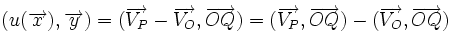
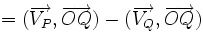 en utilisant l'équiprojectivité du champ V
en utilisant l'équiprojectivité du champ V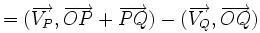
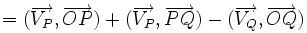
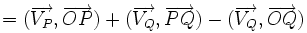 en utilisant de nouveau l'équiprojectivité.
en utilisant de nouveau l'équiprojectivité.
Si on échange les rôles de
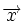 et
et 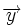 , on obtiendra :
, on obtiendra :On obtient bien :
Linéarité
On déduit de l'antisymétrie que u est linéaire. En effet, pour tout
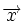 ,
, 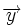 , λ, on a :
, λ, on a :Cette égalité étant vraie pour tout
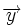 , on en déduit que :
, on en déduit que :On procède de même pour montrer que :
Cas de la dimension 3, torseur
Dans une base orthonormée directe, u, étant un endomorphisme antisymétrique, possède une matrice antisymétrique
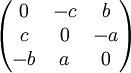
Si on nomme
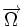 le vecteur de composantes
le vecteur de composantes  , alors la matrice précédente est celle de l'application
, alors la matrice précédente est celle de l'application 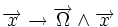 .
.On a donc
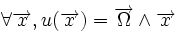 et donc
et donc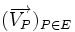 est le champ des moments d'un torseur de résultante
est le champ des moments d'un torseur de résultante 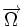 .
.Exemple
L'exemple typique de champ équiprojectif en dimension 3 est le champ des vitesses d'un solide en mouvement. En effet, si P et Q sont deux points du solide, et si on note d la distance entre P et Q, on a :
et en dérivant par rapport au temps :
où
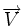 désigne la vitesse en un point.
désigne la vitesse en un point.Le champ des vitesses est donc un torseur. Le vecteur
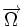 s'appelle vecteur instantané de rotation.
s'appelle vecteur instantané de rotation.Voir aussi
- Portail de la physique
- Portail des mathématiques
Catégories : Algèbre linéaire | Mécanique -
Wikimedia Foundation. 2010.