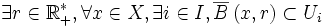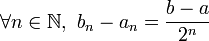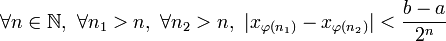- TBW
-
Théorème de Bolzano-Weierstrass
En topologie des espaces métriques, le théorème de Bolzano-Weierstrass donne une caractérisation séquentielle des espaces compacts. Il tire son nom des mathématiciens Bernard Bolzano et Karl Weierstrass.
Sommaire
Énoncé du théorème
Un espace métrique (X,d) est compact (au sens de l'axiome de Borel-Lebesgue) si et seulement si toute suite à valeurs dans X admet une valeur d'adhérence dans X.
Démonstration
Sens direct
On suppose que de tout recouvrement ouvert on peut extraire un sous-recouvrement fini.
Soit
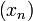 une suite d'éléments de X. Montrons que
une suite d'éléments de X. Montrons que 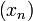 admet une valeur d'adhérence.
admet une valeur d'adhérence.Notons
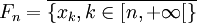 (où
(où 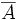 désigne l'adhérence de A).
désigne l'adhérence de A).Posons alors
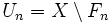 . Si la famille
. Si la famille 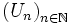 était un recouvrement de X alors par hypothèse on pourrait en extraire un sous recouvrement fini; or
était un recouvrement de X alors par hypothèse on pourrait en extraire un sous recouvrement fini; or 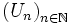 est une famille croissante d'ensembles donc
est une famille croissante d'ensembles donc 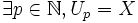 mais alors
mais alors 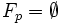 ce qui est exclu car
ce qui est exclu car 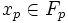 .
.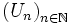 n'est donc pas un recouvrement donc
n'est donc pas un recouvrement donc 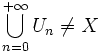 , donc
, donc 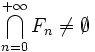
Ce qui montre par définition que
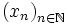 admet une valeur d'adhérence.
admet une valeur d'adhérence.Sens réciproque
Dans cette démonstration, on qualifiera de séquentiellement compact un espace métrique dans lequel toute suite admet une valeur d'adhérence.
Premier lemme (nombres de Lebesgue d'un recouvrement)
Si
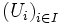 est un recouvrement ouvert d'un espace séquentiellement compact X, alors
est un recouvrement ouvert d'un espace séquentiellement compact X, alors(Où
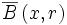 désigne la boule fermée de centre x et de rayon r.)Démonstration
désigne la boule fermée de centre x et de rayon r.)DémonstrationPar l'absurde. on suppose
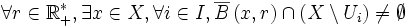 en particulier
en particulier 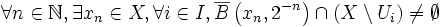 .
.X est séquentiellement compact donc il existe une suite extraite
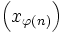 de
de 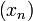 convergeant vers
convergeant vers 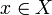 .
.Comme
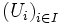 est un recouvrement de X on a
est un recouvrement de X on a 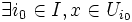 or
or 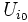 est un ouvert donc
est un ouvert donc 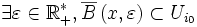 or on peut choisir
or on peut choisir 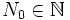 tel que
tel que 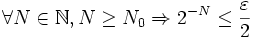 , comme
, comme 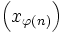 converge vers x il existe
converge vers x il existe 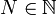 tel que
tel que 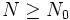 et
et 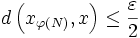 .
.Comme
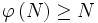 on a
on a 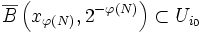 ce qui est absurde.
ce qui est absurde.Deuxième lemme (précompacité)
Si X est un espace métrique séquentiellement compact, alors pour tout nombre
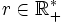 il existe une suite finie de points
il existe une suite finie de points ![\displaystyle \left(x_{k}\right)_{k\in\left[\left[0,n\right]\right]}](/pictures/frwiki/100/d1284f717df6d354d7502bbf62ba8b21.png) de X tel que:
de X tel que: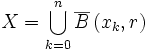 Démonstration
DémonstrationPar l'absurde. On nie le résultat, puis on construit une suite qui nous permettra de trouver une contradiction.
Soit
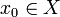 et
et 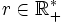 . On suppose construit les termes
. On suppose construit les termes 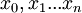 de la suite jusqu'au rang
de la suite jusqu'au rang  tel que
tel que ![\forall\left(i,j\right)\in\left[\left[0,n\right]\right]^{2},i\neq j\Rightarrow d\left(x_{i},x_{j}\right)\geq r](/pictures/frwiki/49/15a77f69b843c8179f1ff8f67f21b625.png) . Par hypothèse on peut choisir
. Par hypothèse on peut choisir 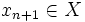 tel que
tel que 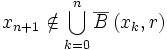 .
.On peut donc construire par récurrence une suite
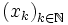 tel que
tel que 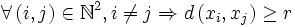 donc
donc 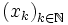 n'admet pas de sous-suite convergente ce qui est en contradiction avec l'hypothèse X est un espace métrique séquentiellement compact.
n'admet pas de sous-suite convergente ce qui est en contradiction avec l'hypothèse X est un espace métrique séquentiellement compact.Fin de la démonstration du théorème
Supposons X séquentiellement compact. Soit
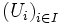 un sous recouvrement ouvert de X.
un sous recouvrement ouvert de X.D'après le premier lemme:
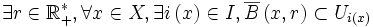 .
.D'après le lemme de précompacité, pour ce r donné il existe une suite finie de points
![\left(x_{k}\right)_{k\in\left[\left[0,n\right]\right]}](/pictures/frwiki/49/14a4d0916afef442adfa32939b6a6b1d.png) de X tel que
de X tel que 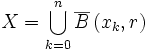 .
.On en déduit donc que la sous-famille
![\left(U_{i\left(x_{k}\right)}\right)_{k\in\left[\left[0,n\right]\right]}](/pictures/frwiki/49/1a31551c0cb1f9795a3ab6d8b3c2e58b.png) recouvre X.
recouvre X.Énoncé dans le cas réel
De toute suite réelle bornée, on peut extraire une sous-suite convergente.
Pour montrer cette propriété, il suffit de remarquer que les intervalles fermés bornés de
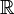 sont compacts (théorème de Borel-Lebesgue). La même propriété s'applique aux suites bornées complexes, ou plus généralement aux suites bornées de vecteurs dans un espace vectoriel normé de dimension finie.Démonstration élémentaire
sont compacts (théorème de Borel-Lebesgue). La même propriété s'applique aux suites bornées complexes, ou plus généralement aux suites bornées de vecteurs dans un espace vectoriel normé de dimension finie.Démonstration élémentaireSoit
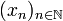 une suite à valeurs dans un segment [a,b] de
une suite à valeurs dans un segment [a,b] de 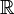 . La démonstration procède en deux temps :
. La démonstration procède en deux temps :-
- 1) Construction de deux suites
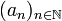 (resp.
(resp. 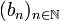 ) croissante (resp. décroissante) telles que l'intervalle [a,b] contient une infinité de termes de la suite
) croissante (resp. décroissante) telles que l'intervalle [a,b] contient une infinité de termes de la suite 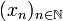 et que
et que
- 2) Construction de la suite extraite.
- 1) Construction de deux suites
- 1) Construction par dichotomie des suites
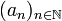 (resp.
(resp. 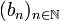 )
)
On définit a0 par a0 = a et b0 par b0 = b.
Pour tout entier naturel n, si l'intervalle
![\left[ a_n , \frac{a_n+b_n}{2} \right]](/pictures/frwiki/49/1de141ae0cc69c56e48b90c7dbaa6d50.png) contient une infinité de termes de la suite
contient une infinité de termes de la suite 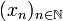 , on pose an + 1 = an et
, on pose an + 1 = an et 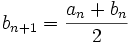 .
.Sinon, l'intervalle
![\left[ \frac{a_n+b_n}{2},b_n \right]](/pictures/frwiki/51/398f5c5488620d8b1749975da22d00d5.png) contient une infinité de termes de la suite
contient une infinité de termes de la suite 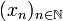 , on pose alors
, on pose alors 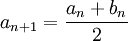 et bn + 1 = bn.
et bn + 1 = bn.On vérifie que ces deux suites ainsi construite sont adjacentes et que l'intervalle [a,b] contient une infinité de termes de la suite
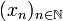 .
.- 2) Construction par récurrence de la suite extraite convergente
Cela revient à dire que nous cherchons une fonction
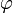 de
de 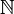 dans
dans 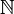 strictement croissante, telle que la suite
strictement croissante, telle que la suite 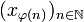 soit convergente. Construisons alors la fonction
soit convergente. Construisons alors la fonction 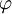 par récurrence.
par récurrence.Posons
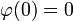 . Pour tout entier n, prenons pour
. Pour tout entier n, prenons pour 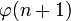 le plus petit entier m strictement supérieur à
le plus petit entier m strictement supérieur à 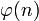 tel que
tel que ![x_m\in \left[ a_{n+1},b_{n+1} \right]](/pictures/frwiki/49/1d5a834ed67d58283932c507bb3870c0.png) (cet entier existe puisque
(cet entier existe puisque ![\left[ a_{n+1}\ ,b_{n+1}\ \right]](/pictures/frwiki/97/afcb3d55f5d8f2dd09bb975887ed1b72.png) contient une infinité de termes de la suite
contient une infinité de termes de la suite 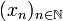 ). La fonction
). La fonction 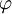 est donc bien définie par récurrence et par construction cette fonction est strictement croissante et vérifie la propriété:
est donc bien définie par récurrence et par construction cette fonction est strictement croissante et vérifie la propriété:Cette propriété nous montre que la suite
 vérifies le critère de Cauchy, elle est donc convergente vers un réel
vérifies le critère de Cauchy, elle est donc convergente vers un réel  appartenant à l'intervalle [a,b].
appartenant à l'intervalle [a,b].Voir aussi
- Portail des mathématiques
Catégories : Théorème de mathématiques | Compacité | Suite
Wikimedia Foundation. 2010.